Javier 12 Feb 2019

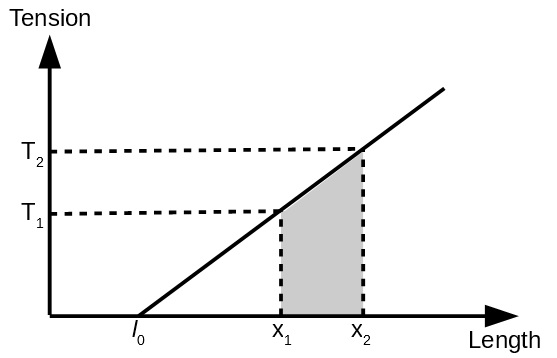
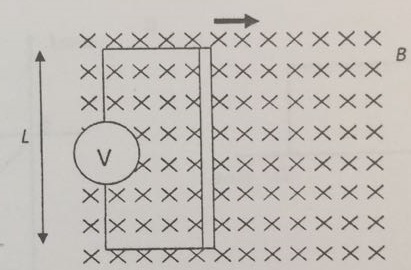
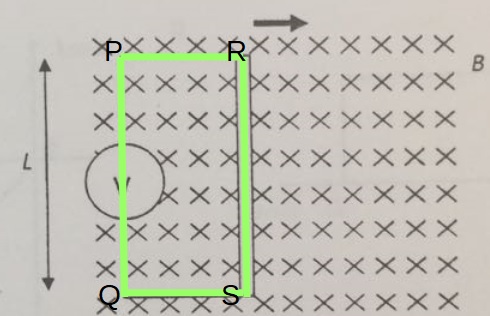
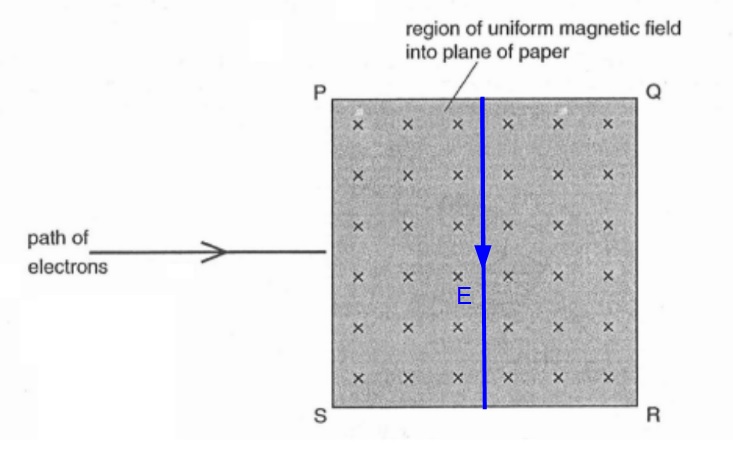
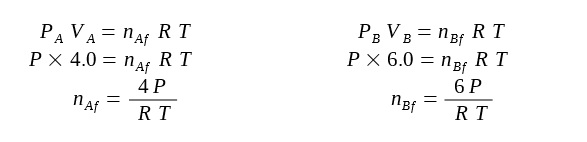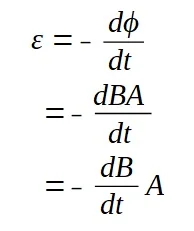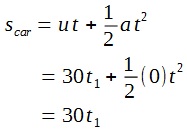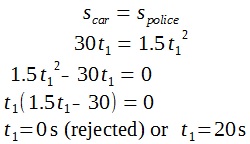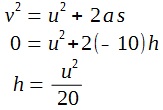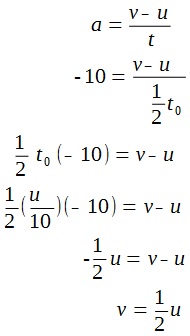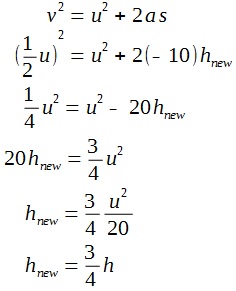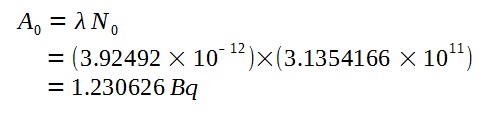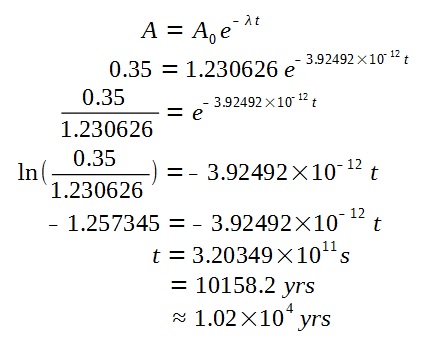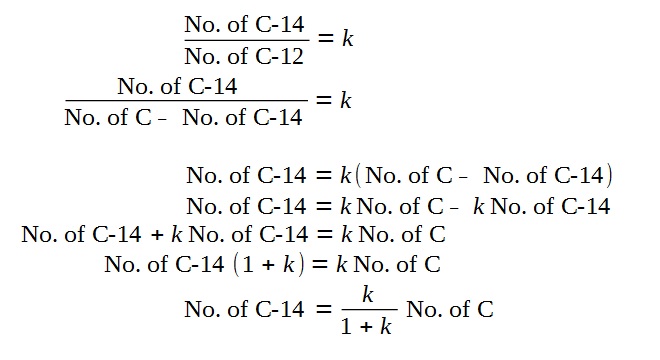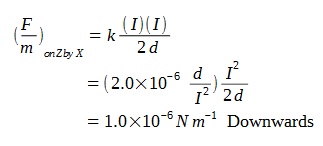Question

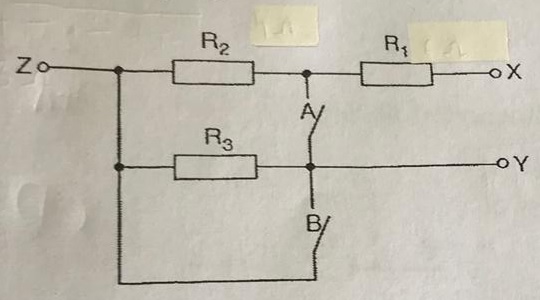
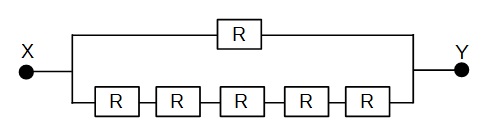
A circuit consists of three resistors, R₁, R₂ and R₃, and two switches A and B, as shown in the figure.

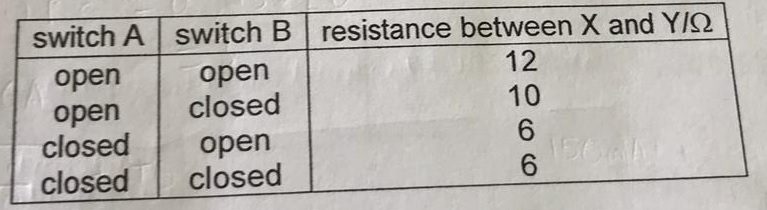
The resistances between terminals X and Y is measured for different settings of the switches A and B. The results are shown in the table.

a) Determine the resistance of resistors R₁, R₂ and R₃.

b) Switch A is now closed and switch B is open. Calculate the resistance between terminals X and Z.

---

Answer

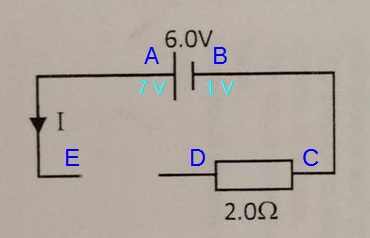
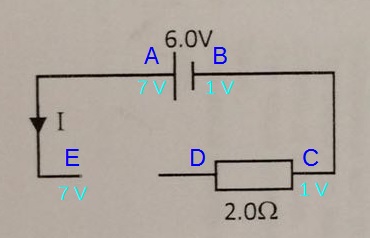
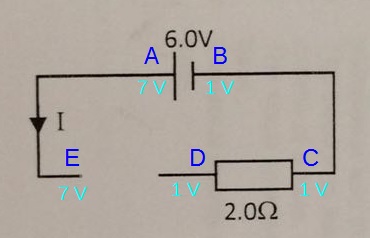
a) From the first row in the table, when switches A and B are both open, the total resistance is 12Ω.

Assuming that X is positive and Y is negative, current will flow through the circuit as shown.

Hence, we see that:

From the second row in the table, when switch A is open and B is closed, the total resistance is 10Ω.

Assuming that X is positive and Y is negative, current will flow through the circuit as shown.

Hence, we see that:

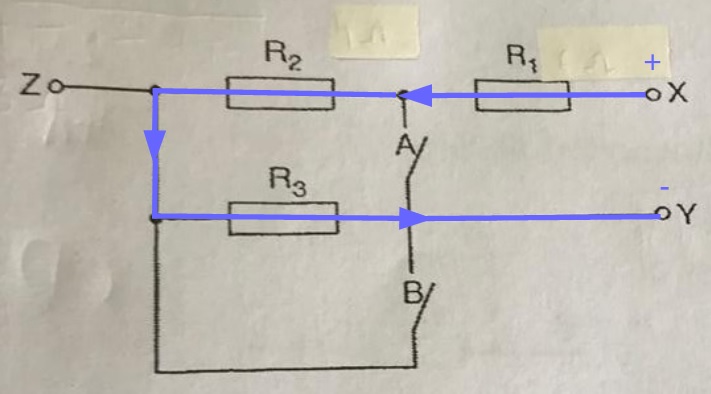
From the third row in the table, when switch A is closed and B is open, the total resistance is 6Ω.

Assuming that X is positive and Y is negative, current will flow through the circuit as shown.

Hence, we see that:

Solving the equations:

we obtain

R2 = 4 Ω

R3 = 2 Ω

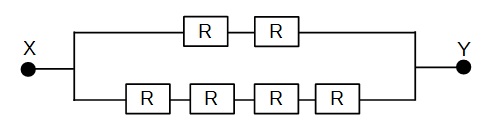
b) When switch A is closed and B is open, we need to find the total resistance between X and Z.

Assuming that X is positive and Z is negative, current will flow through the circuit as shown.

Maeen 5 Jul 2018

Question

A lamp is placed 30 cm above a metal surface which contains atoms of diameter 20 x 10^-10 m. The lamp can be considered as a point source with power 0.015 W. It may be assumed that each electron can collect energy from a circular area which has a radius equal to that of the atom

a i) Find the intensity of the electromagnetic radiation directed on the atom.

ii) Hence, calculate the power incident on each atom.

iii) Determine, on the basis of wave theory, the time required for an electron to collect sufficient energy to be emitted from the metal if the work function of the metal is 3.2 x 10^-19 J

iv) Comment on this calculation with actual experimental data and conclude the validity of wave theory.

---

Answer

a i) If we treat the lamp as a point source that emits light in all directions (3D),

Note that the area used  is the total area which the power of the lamp is spread over.

aii) Now that we know the intensity of the light incident on the atom, we can calculate the power incident on the atom.

Note that the area used is the area of the atom which absorbs the power.

a iii) Since the electron requires a certain amount of energy to be emitted, we can calculate the time needed.

a iv) This calculation shows that if light were to be treated as a wave, the electron will required 7.68s to collect enough energy to be emitted. However, the experimental data shows that electrons will be emitted almost immediately, with a time much less than 7.68s.

This means that the wave theory cannot be used to describe the photoelectric effect.

Maeen 20 Apr 2018

Question

A breathing monitor consists of an 8-turn coil attached to a patient’s chest. As a breath is inhaled, the area of the coil varies from 0.120 m² to 0.124 m². The magnetic flux density of the Earth is 50 x 10^-6 T and makes an angle of 22.5^o with the axis of the coil.

If the patient inhales for 1.59s, what is the average emf induced in the coil during the inhalation?

A) 0.116 μV

B) 0.126 μV

C) 0.930 μV

D) 1.01 μV

---

Answer

To calculate the induced emf, the magnetic flux linkage of the coil has to be calculated.

Before inhalation,

After inhalation,

The induced emf can now be calculated.

Answer is C

Maeen 16 Apr 2018

Question

A displacement against position graph for a longitudinal wave is shown below. Which points represents the compressions and rarefactions?

---

Answer

For the graph, the position axis represents the location of the equilibrium position of a particle. The displacement axis gives the displacement of the particle from its equilibrium position. For this question, we assume that positive displacement is towards the right.

For example, in the graph below, Particle A has a equilibrium position at 0.80 m and it is displaced 1.5 mm to the right of its equilibrium position.

We can show the displacement on the graph:

The displacements of B1, B2, B3, C1, C2 and C3 are shown.

Hence, B2 is the compression and C2 is the rarefaction.

Shi Qi 4 Mar 2018 - 2

Question

In two widely-separated planetary systems whose suns have masses S₁ and S₂, planet P₁ of mass M₁ and planet P₂ of mass M₂ are observed to have circular orbits of equal radii respectively. If P₁ completes and orbit in half the time by P₂, it may be deduced that

A) S₁ = S₂ and M₁ = 0.25 M₂

B) S₁ = 4 S₂ only

C) S₁ = 4 S₂ and M₁ = M₂

D) S₁ = 0.25 S₂ only

E) S₁ = 0.25 S₂ and M₁ = M₂

---

Answer

For the system with S₁ and P₁, the gravitational force on P₁ provides the centripetal force.

Similarly for the system with S₂ and P₂,

Since the period of P₁ is half of P₁,

Since the periods do not depend on the masses of the planets, we can only deduce that S₁ = 4 S₂.

Hence, answer is B.

Shi Qi 4 Mar 2018 - 1

Question

At a point outside the Earth and a distance of x from its centre, the Earth’s gravitational field strength is 5 N kg^-1. At the Earth’s surface, the field strength is about 10 N kg^-1. Which of the following gives an approximate value for the radius of the Earth?

A) x/5

B) x/2√2

C) x√2

D) x/√2

---

Answer

At point x, the gravitational field strength is given by the formula:

At the surface of the Earth, the gravitational field strength is given by the formula:

Since g_s ≈ 2 g_x,

Hence, the answer is D.

Shi Qi 20 Feb 2018

Question

A wire that obeys Hooke’s Law of length x₁ when it is in equilibrium under tension T₁. It’s length becomes x₂ when the tension is increased to T₂. What is the extra energy stored in the wire as a result of this process?

A) 1/4 ( T2 + T1 ) ( x2 - x1 )

B) 1/4 ( T2 + T1 ) ( x2 + x1 )

C) 1/2 ( T2 + T1 ) ( x2 - x1 )

D) 1/2 ( T2 + T1 ) ( x2 + x1 )

E) ( T2 - T1 ) ( x2 - x1 )

---

Answer

The extra energy is stored as elastic potential energy.

The graph of tension against total length is shown below. The extra elastic potential  energy is given by the shaded area.

Answer is C.

Shi Qi 2 Oct 2017

Question

A metal nugget floats in between some water and mercury. The densities of the metal nugget, mercury and water are 7900 kg m^-3, 13600 kg m^-3 and 1000 kg m^-3 respectively.

What is the ratio of the volume of the nugget submerged in water to that of mercury?

A) 0.541
B) 0.826
C) 0.924
D) 1.21

---

Answer

Since the nugget is suspended in the liquids, the weight of the nugget is equal to the upthrust.

The nugget displaces both water and mercury, hence, the upthrust is due to the both the weight of the water displaced and the weight of the mercury displaced.

The volume of the nugget is the sum of the volume of water displaced and the volume of mercury displaced.

The answer is B.

Shi Qi 22 Sep 2017

Question

Two containers of volume 4.0m³ and 6.0m³ contain an ideal gas at pressures of 100Pa and 50Pa respectively. Their temperatures are equal. They are joined by a tube of negligible volume. The gas flows from one container to the other with no change in temperature. The final pressure will be

A) 70 Pa
B) 75 Pa
C) 80 Pa
D) 150 Pa

---

Answer

Before the valve is opened, we first find an expression for the initial number of moles of gas in each container:

Now, the total initial number of moles of gas can be found:

After the valve is opened, the pressure in each container is now the same and the temperature remains the same.

The new expression for the final number of moles in each container is found again:

Now, the total final number of moles of gas can be found:

Since the total number of moles remains the same,

Answer is A.

Brendan 4 Jun 2017 - 2

Question

A copper bar of length L is moving to the right with a uniform speed v in a region of uniform magnetic field of flux density B, directly perpendicularly downwards into the paper in the figure below.

The ends of the rods are rigidly connected to a voltmeter which moves with the rod. What is the reading on the voltmeter?

A) zero
B) non-zero reading less than Blv
C) BLv
D) more than BLv

---

Answer

Method 1:

Consider wires PQ and RS. Since both wires are moving through the magnetic field, both of them will have an induced e.m.f. of BLv.  

Based on Fleming’s Right Hand rule, the induced current of PQ and RS will both be upwards.

This means that the resultant current will be zero and the e.m.f. measured by the voltmeter will be zero.

Answer is A.

---

Method 2:

Consider the closed loop PQRS. As the loop moves through the magnetic field, the magnetic flux linkage remains the same since the area of the loop does not change.

Since there is no change in the magnetic flux linkage, by Faraday’s law there is no induced e.m.f. and the voltmeter will read 0 V.

Answer is A.

Brendan 4 Jun 2017 - 1

Question

An e.m.f. is induced in a coil placed in a changing magnetic field. The flux density B of this field varies with time as shown below.

At which value of t is the magnitude of the e.m.f. induced in the wire a maximum?

A) 1 ms
B) 2 ms
C) 3 ms
D) 4 ms

---

Answer

The induced e.m.f. is given by the formula:

Hence the magnitude of the induced e.m.f. is given by:

This means that the induced e.m.f. will be the greatest when the rate of change of the magnetic flux density is the greatest.

From the graph, the rate of change of the magnetic flux density is the greatest when the gradient of the graph is the greatest.

This means that the induced e.m.f. will be the greatest at time t = 0 ms and t = 4 ms.

Hence the answer is D.

James 27 Feb 2017 - 2

Question

A car travelling at a constant speed of 30 ms_-1 passes a police car, which is at rest.

The police officer accelerates at a constant rate of 3.0 ms_-2 and maintains this rate of acceleration until he pulls next to the speeding car. Assume that the police car starts to move at the moment the car moves past the police car.

What is the time required for the police officer to catch up with the car?

---

Answer

We assume that the police car catches up with the car at time t₁.

The following formula for displacement can be obtained:

When the police car catches up with the car, both have travelled the same displacement.

The police car requires 20 s to catch up with the car.

James 27 Feb 2017 - 1

Question

A body is thrown vertically up from the ground with an initial speed u and it reaches the maximum height h at time t₀.

What is the height it reaches at ½ t₀?

---

Answer

We first define the upwards direction as positive.

When the body reaches the maximum height, the speed of the body is 0.

Knowing this, we can find expressions for h and t₀. The acceleration of the body is taken to be 10 ms^-2 downwards. Since we define upwards to be positive, the acceleleration will be -10 ms^-2.

When the time is ½ t₀,

Now that we know the speed at ½ t₀, we can find the height travelled by the body.

James 29 Sep 2016

Question

Find the potential difference across the 2.0 Ω resistor.

Find the potential difference across the open gap in the circuit.

---

Answer

The easiest way to find the potential difference in this case is to assign values of potential at the various points.

We first start at the sides of the cell, A and B.

We can assign any value as long as the difference between A and B is 6.0 V and A is a higher value than B.

In this case, we choose A to be 7 V and B to be 1 V.

All connecting wires are assumed to have no resistance. This means that the value of potential at all points of the same wire are of the same value.

Hence, E has a value of 7 V and C has a value of 1 V.

Since the circuit is an open circuit and no current flows, from V = I R, the potential difference across the 2.0 Ω resistor is zero.

This means that the values of the potential at both sides of the resistor are the same.

From the diagram, we can see that the difference between the potential at C and D is 0 V.

Hence, the potential difference across the 2.0 Ω resistor is zero.

From the diagram, we can see that the difference between the potential at E and D is 6 V.

Hence, the potential difference across the open gap in the circuit is 6.0 V.

Yusong 25 Aug 2016

Question

The figure shows a long horizontal wire PQ carrying a steady current of 50A in the direction QP. A copper wire RS of diameter 0.40mm hangs horizontally at a distance of 0.15m below wire PQ using some threads.

A current flows through RS such that there is no tension in the threads.

Identify the direction is the current and draw a free body diagram of the forces acting on RS.

---

Answer

If the current in RS is flowing opposite to the current in PQ, the two wires will repel each other.

Since the wire is suspended in equilibrium, the resultant force must be zero.

F_up = F_down
T₁ + T₂ = F_B + W

We can see that it is not possible for the tension to be zero.

If the current in RS is flowing in the same direction as the current in PQ, the two wires will repel attract other.

Since the wire is suspended, the resultant force must be zero.

F_up = F_down
T₁ + T₂ + F_B = W

When the tensions in both threads are zero, we have

F_B = W

Hence, current in RS must be flowing in the same direction as PQ, in the direction SR.

The free body diagram when there is no tension in the threads is as shown below:

Jia Yee 25 Aug 2016

Question

The figure below shows a region PQRS of a uniform magnetic field directed downwards into the plane of the paper.

Electrons, all having the same speed, enter the region of the magnetic field.

a) On the figure, show the path of the electrons as they pass through the magnetic field emerging from side QR.

b) A uniform electric field is also applied in the region PQRS so that the electrons now pass undeflected through this region. On the figure, mark with an arrow labelled E, the direction of the electric field.

c) The undeflected electrons in (b) each have charge -e, mass m and speed v. State and explain the effect if any, on the particles entering the region PQRS of the same magnetic and electric fields in (b) if the particles each have

i) charge -e, mass m and speed 2v.

ii) charge +e, mass m and speed v.

---

Answer

a) Since the electrons are moving to the right, it is the same as a current flowing to the left. By using Flemming’s left hand rule, the magnetic force on the electrons is downwards.

According to Flemming’s left hand rule, the magnetic force will always be perpendicular to the velocity of the electrons. This means that it provides a centripetal force. Hence, the electrons will move in a circular path as shown.

Once the electrons exit the magnetic field, they will move in a straight path since there is no resultant force acting on the electrons. We ignore the effects of the weight because the acceleration of due to the weight is too small to cause any significant change in the velocity of the electrons.

b) In order for the electrons to pass through undeflected, the resultant force on the electrons must be zero. Since the magnetic force on the electrons is downwards, the electric force acting on the electrons must be of the same magnitude and acting upwards.

In order for the electric force to be acting upwards, electric field lines must be directed downwards. (Since electrons are negatively-charged, they will experience an electric force in the opposite direction to the electric field lines.)

c)  When an electron is moving with a speed of v, it passes straight through without any deflection. The magnetic force and electric forces on the electrons are given by the following formulae:

F_B= Bqv
F_E = qE

Since the downwards magnetic force is now greater than the upwards electric force, the resultant force is downwards and the particle will curve downwards as it passes through the region.

ii) When a particle of the opposite charge (+e) and mass (m) and same speed (2v) moves through the field, the directions of the magnetic force and electric forces are now reversed.

The electric force acts downwards and the magnetic force acts upwards on the particle.

However, both forces are still of the same magnitude and the resultant force is still zero. Hence, the particle will still pass through the region undeflected.

Farhan 05 Aug 2016

Question

A piece of furniture was recovered from an archaeological site and carbon dating was used to estimate its age. It was assumed that the proportion of Carbon-14 to natural carbon (Carbon-12) in living wood was 1.25 x 10^-12. If the number of particles emitted from 5 g of carbon prepared from the specimen was 21 per minute, how old is the specimen?
[The half-life of Carbon-14 is 5600 years and the mass number of natural carbon is 12.]

---

Answer

Since the proportion of Carbon-14 is very small compared to Carbon-12, we can assume for the sake of finding the number of carbon atoms that all of the carbon in the specimen is Carbon-12.

Given that the proportion of Carbon-14 to Carbon-12, we can find the original number of Carbon-14 atoms when the piece of furniture was living wood using the following approximation. (Refer to bottom for further discussion of this approximation.)

Using the half-life, we can calculate the decay constant of Carbon-14.

Hence, we can find the initial activity when the specimen was still living wood.

The final activity of the specimen is given by

Now, we can find the age of the specimen.

---

Discussion on Approximation

Based on the phrasing of the question that

“the proportion of Carbon-14 to natural carbon is k”,

However, since the k is small compared to 1,

Hence, we arrive at

Yusong 04 Aug 2016

Question

A simple iron-core transformer is shown below:

Suggest why thermal energy is generated in the core when the transformer is in use.

---

Answer

Consider a section of the transformer core which is coloured blue as shown below:

As the magnetic field produced by the current in the primary coil increases, the magnetic flux linkage of the section of the coil will increase.

By Faraday’s Law, there will be an induced e.m.f. produced in the section of the core. By Lenz’s Law, there will be a current flowing in the section of the core which will produce a magnetic effect to oppose the change.

By the Right Hand Grip Rule, this means that a circular current must be flowing in the section of the core in order to produce the magnetic field. (This circular current is called the eddy current.)

Since an induced current is flowing in the core, by P = I ² R, there will be power dissipated in the core and thermal energy is generated in the core of the transfomer.

Minqi 19 May 2016 - 4

Question

A set-up to control the brightness of a bulb is shown below.

X and Y are to connected to a uniform circular ring which has a constant cross-sectional area and resistivity X is connected to the left side of the ring.

Where should Y be connected for the light to be the dimmest?

---

Answer

The circular ring and its connection point can be seen as a connection of 6 resistors of resistance R as shown below:

When Y is connected to A, the connection is as shown below:

The effective resistance is 0.833 R.

When Y is connected to B, the connection is as shown below:

The effective resistance is 1.33 R.

When Y is connected to C, the connection is as shown below:

The effective resistance is 1.5 R.

When Y is connected to D, the connection is as shown below:

The effective resistance is 1.33 R.

Hence, connecting Y to C will result in the greatest resistance and the light bulb will be the dimmest.

Minqi 19 May 2016 - 3

Question

The diagram below shows three parallel wires X, Y and Z placed in a horizontal plane. Wires X and Z carry current I in opposite directions. Wire Y carries a current of 3I in the direction shown and is equidistant from the other wires.

The magnitude of the force per unit length between two parallel wires placed a distance apart, each carrying a current of I is 2.0 x 10-6 N m-1. What is the direction and magnitude of the net force per unit length acting on the wire Z?

(The force per unit length is proportional to the product of the currents in the two wires and inversely proportional to the separation between them.)

---

Answer

Based on the information regarding the forces per unit length between two wires,

\( {F \over m} = k {I_1 I_2 \over r} \)

Based on the value of the force given,

The force between Y and Z is attractive because the currents in both wires are flowing in the same direction.

The force between X and Z is repulsive because the currents in both wires are flowing in the opposite directions.

Hence, the resultant force per unit length is 5.0 x 10^-6 N m^-1 upwards towards Y.